 |
|||||
| Home | Research | For Teachers | HISTORY Level 1 Level 2 Level 3 |
PRINCIPLES Level 1 Level 2 Level 3 |
CAREER Level 1 Level 2 Level 3 |
| Gallery | Hot Links | What's New! | |||
| Web Administration and Tools | |||||
|
|||||
| Home | Research | For Teachers | HISTORY Level 1 Level 2 Level 3 |
PRINCIPLES Level 1 Level 2 Level 3 |
CAREER Level 1 Level 2 Level 3 |
| Gallery | Hot Links | What's New! | |||
| Web Administration and Tools | |||||
A rocket in
its simplest form is a chamber enclosing a gas under pressure. A small opening at one end
of the chamber allows the gas to escape, and in doing so provides a thrust that propels
the rocket in the opposite direction. A good example of this is a balloon. Air inside a
balloon is compressed by the balloon's rubber walls. The air pushes back so that the
inward and outward pressing forces are balanced. When the nozzle is released, air escapes
through it and the balloon is propelled in the opposite direction.
When we think of rockets, we rarely think of balloons. Instead, our attention is drawn to the giant vehicles that carry satellites into orbit and spacecraft to the Moon and planets. Nevertheless, there is a strong similarity between the two. The only significant difference is the way the pressurized gas is produced. With space rockets, the gas is produced by burning propellants that can be solid or liquid in form or a combination of the two.
One of the interesting facts about the historical development of rockets is that while rockets and rocket-powered devices have been in use for more than two thousand years, it has been only in the last three hundred years that rocket experimenters have had a scientific basis for understanding how they work.
The science of rocketry began with the publishing of a book in 1687 by the great English scientist Sir Isaac Newton. His book, entitled Philosophiae Naturalis Principia Mathematica, described physical principles in nature. Today, Newton's work is usually just called the Principia. In the Principia, Newton stated three important scientific principles that govern the motion of all objects, whether on Earth or in space. Knowing these principles, now called Newton's Laws of Motion, rocketeers have been able to construct the modern giant rockets of the 20th century such as the Saturn V and the Space Shuttle. Here now, in simple form, are Newton's Laws of Motion.
As will be explained shortly, all three laws are really simple statements of how things move. But with them, precise determinations of rocket performance can be made.
This law of motion is just an obvious statement of fact, but to know what it means, it is necessary to understand the terms rest, motion, and unbalanced force.
Rest and motion can be thought of as being opposite to each other. Rest is the state of an object when it is not changing position in relation to its surroundings. If you are sitting still in a chair, you can be said to be at rest. This term, however, is relative. Your chair may actually be one of many seats on a speeding airplane. The important thing to remember here is that you are not moving in relation to your immediate surroundings (the plane), however you and the plane are moving relative to the earth. If rest were defined as a total absence of motion, it would not exist in nature. Even if you were sitting in your chair at home, you would still be moving, because your chair is actually sitting on the surface of a spinning planet that is orbiting a star. The star is moving through a rotating galaxy that is, itself, moving through the universe. While sitting "still," you are, in fact, traveling at a speed of hundreds of kilometers per second. You can define accelerations and velocities in the following manner
v(chair-absolute) = v(chair-relative
to the plane) + v(plane-absolute)
a(chair-absolute) = a(chair-relative to the plane) + a(plane-absolute)
where v(plane-absolute) and a(plane-absolute) represent the velocity and acceleration, respectively, of the plane in relation to the ground, which is considered a "fixed" or inertial frame of reference.
Motion is also a relative term. All matter in the universe is moving all the time, but in the first law, motion here means changing position in relation to surroundings. A ball is at rest if it is sitting on the ground. The ball is in motion if it is rolling. A rolling ball changes its position in relation to its surroundings. When you are sitting on a chair in an airplane, you are at rest, but if you get up and walk down the aisle, you are in motion. A rocket blasting off the launch pad changes from a state of rest to a state of motion.
The third term important to understanding this law is unbalanced force. If you hold a ball in your hand and keep it still, the ball is at rest. All the time the ball is held there though, it is being acted upon by forces (weight, W, and the force exerted by your hand on the ball, N). The force of gravity is trying to pull the ball downward, while at the same time your hand is pushing against the ball to hold it up. The forces acting on the ball are balanced. Let the ball go, or move your hand upward, and the forces become unbalanced. The ball then changes from a state of rest to a state of motion.
In rocket flight, forces become balanced and unbalanced all the time. A rocket sitting on the launch pad is balanced. The surface of the pad pushes the rocket up while gravity tries to pull it down. As the engines are ignited, the thrust from the rocket unbalances the forces, and the rocket travels upward. Later, when the rocket runs out of fuel, it slows down, stops at the highest point of its flight, then falls back to Earth.
Objects in space also react to forces. A spacecraft moving through the solar system is in constant motion. The spacecraft will travel in a straight line if the forces on it are in balance. This happens only when the spacecraft is very far from any large gravity source such as Earth or the other planets and their moons. If the spacecraft comes near a large body in space, the gravity of that body will unbalance the forces and curve the path of the spacecraft. This happens, in particular, when a satellite is sent by a rocket on a path that is parallel to Earth's surface. If the rocket shoots the spacecraft fast enough, the spacecraft will orbit Earth. As long as another unbalanced force, such as friction with gas molecules in orbit or the firing of a rocket engine in the opposite direction from its movement, does not slow the spacecraft, it will orbit Earth forever.
Now that the three major terms of this first law have been explained, it is possible to restate this law. If an object, such as a rocket, is at rest, it takes an unbalanced force to make it move. If the object is already moving, it takes an unbalanced force, to stop it, change its direction from a straight line path, or alter its speed.
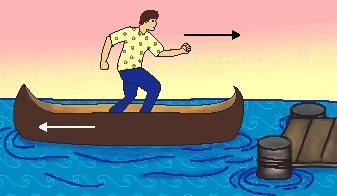
For the time being, we will skip the second law and go directly to the third. This law states that every action has an equal and opposite reaction. If you have ever stepped off a small boat that has not been properly tied to a pier, you will know exactly what this law means.
Press
to see
Animation
A rocket can lift off from a launch pad only when it expels gas out of its engine. The rocket pushes on the gas, and the gas in turn pushes on the rocket. The whole process is very similar to riding a skateboard. Imagine that a skateboard and rider are in a state of rest (not moving). The rider jumps off the skateboard. In the third law, the jumping is called an action. The skateboard responds to that action by traveling some distance in the opposite direction. The skateboard's opposite motion is called a reaction. When the distance traveled by the rider and the skateboard are compared, it would appear that the skateboard has had a much greater reaction than the action of the rider. This is not the case. The reason the skateboard has traveled farther is that it has less mass than the rider. This concept will be better explained in a discussion of the second law.
With rockets, the action is the expelling of gas out of the engine. The reaction is the movement of the rocket in the opposite direction. To enable a rocket to lift off from the launch pad, the action, or thrust, from the engine must be greater than the weight and drag of the rocket. In space, however, even tiny thrusts will cause the rocket to change direction.
One of the most commonly asked questions about rockets is how they can work in space where there is no air for them to push against. The answer to this question comes from the third law. Imagine the skateboard again. On the ground, the only part air plays in the motions of the rider and the skateboard is to slow them down. Moving through the air causes friction, or as scientists call it, drag. The surrounding air impedes the action-reaction.
As a result rockets actually work better in space than they do in air. As the exhaust gas leaves the rocket engine it must push away the surrounding air; this uses up some of the energy of the rocket. In space, the exhaust gases can escape freely.
This law of motion is essentially an empirically derived statement of a mathematical equation. The three parts of the equation are mass (m), acceleration (a), and force (f), if the mass remains constant. Using letters to symbolize each part, the equation can be written as follows:
By using simple algebra, we can also write the equation two other ways:
The first version of the equation is the one most commonly referred to when talking about Newton's second law. It reads: force equals mass times acceleration. To explain this law, we will use an old style cannon as an example.
When the cannon is fired, an explosion propels a cannon ball out the open end of the barrel. It flies a kilometer or two to its target. At the same time the cannon itself is pushed backward a meter or two. This is action and reaction at work (third law). The force acting on the cannon and the ball is the same. What happens to the cannon and the ball is determined by the second law. Look at the two equations below.
The first equation refers to the cannon and the second to the cannon ball. In the first equation, the mass is the cannon itself and the acceleration is the movement of the cannon. In the second equation the mass is the cannon ball and the acceleration is its movement. Because the force (exploding gun powder) is the same for the two equations, the equations can be combined and rewritten below.
In order to keep the two sides of the equations equal, the accelerations vary inversely with mass. In other words, the cannon has a large mass and a small acceleration. The cannon ball has a small mass and a large acceleration.
Let's apply this principle to a rocket. Replace the mass of the cannon ball with the mass of the gases being ejected out of the rocket engine. Replace the mass of the cannon with the mass of the rocket moving in the other direction. Force is the pressure created by the controlled explosion taking place inside the rocket's engines. That pressure accelerates the gas one way and the rocket the other.
Some interesting things happen with rockets that don't happen with the cannon and ball in this example. With the cannon and cannon ball, the thrust lasts for just a moment. The thrust for the rocket continues as long as its engines are firing. Furthermore, the mass of the rocket changes during flight. Its mass is the sum of all its parts. Rocket parts includes engines, propellant tanks, payload, control system, and propellants. By far, the largest part of the rocket's mass is its propellants. But that amount constantly changes as the engines fire. That means that the rocket's mass gets smaller during flight. In order for the left side of our equation to remain in balance with the right side, acceleration of the rocket has to increase as its mass decreases. That is why a rocket starts off moving slowly and goes faster and faster as it climbs into space.
Newton's second law of motion is especiaily useful when designing efficient rockets. To enable a rocket to climb into low Earth orbit, it is necessary to achieve a speed, in excess of 28,000 km per hour. A speed of over 40,250 km per hour, called escape velocity, enables a rocket to leave Earth and travel out into deep space. Attaining space flight speeds requires the rocket engine to achieve the greatest action force possible in the shortest time. In other words, the engine must burn a large mass of fuel and push the resulting gas out of the engine as rapidly as possible. Ways of doing this will be described in the next chapter, practical rocketry..
Newton's second law of motion can be restated in the following way: the greater the mass of rocket fuel burned, and the faster the gas produced can escape the engine, the greater the thrust of the rocket.
An unbalanced force must be exerted for a rocket to lift off from a launch pad or for a
craft in space to change speed or direction (first law). The amount of thrust (force)
produced by a rocket engine will be determined by the mass of rocket fuel that is burned
and how fast the gas escapes the rocket (second law). The reaction, or motion, of the
rocket is equal to and in the opposite direction of the action, or thrust, from the engine
(third law).
TO THE PRACTICAL ROCKETRY PAGE - SECTION 2
Send all comments to 
aeromaster@eng.fiu.edu
© 1995-98 ALLSTAR Network. All rights reserved worldwide.
| Funded in part by |  |
Updated: February 23, 1999