Home	Research	For Teachers	HISTORY Level 1 Level 2 Level 3	PRINCIPLES Level 1 Level 2 Level 3	CAREER Level 1 Level 2 Level 3
Gallery	Hot Links	What's New!
Web Administration and Tools

Section 1.13 - Hydraulic System Hand Pumps

Functions

1. Hydraulic system hand pumps are used to test the hydraulic system when the plane is on the ground; and,
2. acts as an Emergency system of power

Principles of Operation

The hand pump converts the power of a human being to hydraulic horsepower. For this reason, such pumps are used in older model aircraft.

Types

There are several types of handpumps.

1. Single action - single impulse (S.A.S.I.)
2. Double action - double impulse (D.A.D.I.)
3. Single action - double impulse (S.A.D.I.)

Analysis of a S.A.S.I. Pump

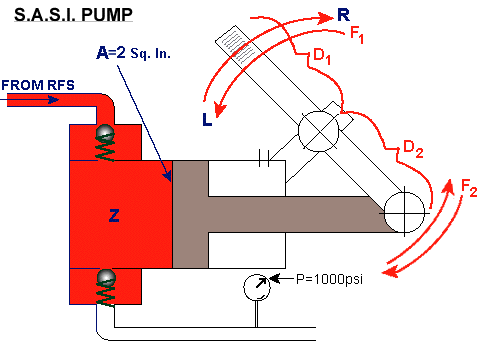
The pump works on the principle of mechanical advantage. 
When pilot moves the handle away to L (see diagram below), a low pressure is caused to form in the chamber of the pump, Z. Since the reservoir liquid pressure is greater than the pressure in Z, liquid is forced around the check valve from the reservoir line. When the pump handle is moved to R, a positive pressure will form in Z, causing the check valve to the reservoir side to close. Since the hydraulic system liquid is incompressible, it is forced out through the bottom check valve to the system line.

Suppose the force delivered by the pilot was F₁ = 100 lb, at a distance D₁ = 20 inches from the pump handle pivot, and suppose that D₂ = 1 inch from the pivot to the pump piston.  Then by moment equilibrium (F₁D₁=F₂D₂), the force acting on the piston would be F₂ = 2000 lb. If the piston area on which the oil acts is A = 2 square inches, then the pressure developed is

The volumetric output . In this case, . But a man’s average output is about 50 lb for F₁. Therefore, the piston area must be made half its present size (if the pressure is to remain constant). Thus, volumetric output will then decrease to 1 cu. in., since .

Problem: (SASI Pump)
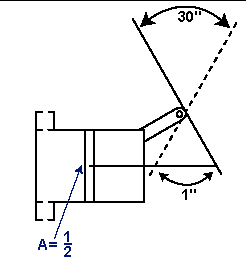Given a SASI pump connected to a hydraulic system under 3000 psi pressure and the mechanical advantage of the pump (the ratio of D₁ to D₂ ) to be 30 to 1, find:
1)  the number of cycles needed to pump 300 cubic inches of fluid into the system; and,
2)  the force to be applied at the pump handle. Assume that the piston surface area is 0.5 square inches.

Since the system pressure = 3000 psi and this acts on a surface area of 1/2 square inch, the force generated by the hydraulic fluid is 1500 lbs.

Using the mechanical advantage of 30 to 1, we find that the force at the pump handle will be

or .

Since the piston stroke is D₂, the volume moved per cycle is D₂ times the piston surface area

or

Thus, per cycle, 0.5 cubic inches of fluid is pumped into the system.

The number of cycles needed to pump 300 cubic inches of fluid may be found from

then

Analysis of a D.A.D.I. Pump

The DADI pump works in the same way that the SASI pump works, except that while chamber A is filling up from the reservoir, chamber B is pumping oil to the system.

Analysis of a S.A.D.I. Pump

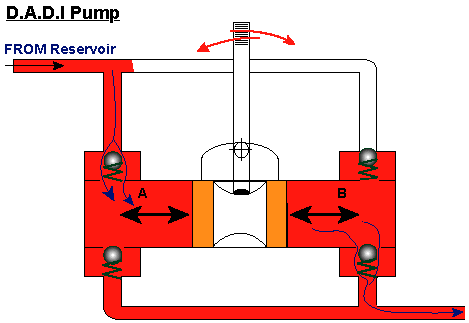
As the pump handle pulls the piston to the left, the oil trapped in A is pushed out through check valve 1 to the system. At the same time, a low pressure is created in B and ball 2 opens, letting in oil. As the pump handle pushes the piston to the right, a positive pressure is created in B which closes check valve 2. But, since oil in B is trapped, it escapes to side A when the pressure is great enough to open check valve 3. Now that the oil is in side A, the pressure on that side is still great enough to open check valve 1.

Send all comments to aeromaster@eng.fiu.edu
© 1995-98 ALLSTAR Network. All rights reserved worldwide.

Funded in part by

Updated: February 17, 1999