
Home	Research	For Teachers	HISTORY Level 1 Level 2 Level 3	PRINCIPLES Level 1 Level 2 Level 3	CAREER Level 1 Level 2 Level 3
Gallery	Hot Links	What's New!
Web Administration and Tools

Aeronautics - Fluid Dynamics - Level 3

Incompressible flow around a cylinder and a wing section

Netscape users: Please be advised that this page contains JAVA script to show the animations. In order to view the animations effectively, please move your mouse over the "hot" words embedded in the text but do NOT click on them, otherwise it will take you back to our homepage. If that happens, use the "BACK" button to return to this page.

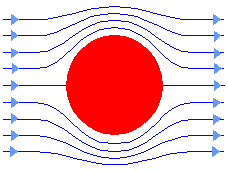
Incompressible flow around a stationary cylinder:

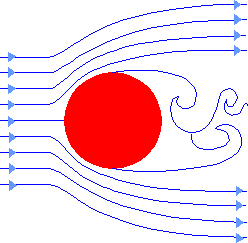
Inviscid (ideal) flow:	Viscous flow:

Inviscid (Ideal) flow around a stationary cylinder:

The impingement of flow on the cylinder creates a stagnation point on the approaching surface. The departure of the flow away from the cylinder creates another stagnation point on the trailing surface.

In the idealized situation where viscosity is neglected, the no-slip condition at the surface of the cylinder does not apply.

Also in the absence of vorticity (inviscid flow) flow separation cannot occur.

Viscous flow around a stationary cylinder:

One stagnation point is created in front of the cylinder.

Because of the viscosity, a no-slip condition exists everywhere on the surface of the cylinder, i.e., the velocity must vanish everywhere on the surface.

Consequently a boundary layer is created where the velocity transitions from a value of zero at the surface to the free stream value some distance away from the cylinder surface.

The inertia of the fluid as it rounds the top and bottom of the trailing surface causes the flow to separate at these locations.

This creates a disturbed wake (Von-Karman vortex street) downstream from the cylinder.

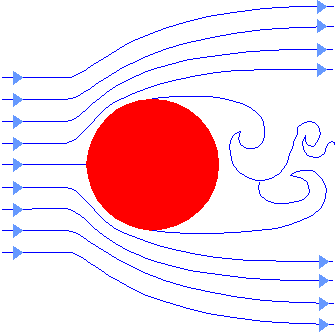Incompressible flow around a stationary wing section:

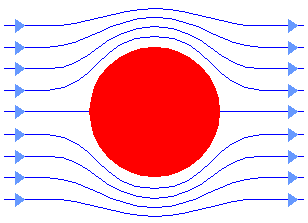
Inviscid (ideal) flow:	Viscous flow:

Inviscid (Ideal) flow around a stationary wing section:

As with the cylinder, stagnation points are created by the impingement of the flow on the approaching surface and the departure of the flow on the trailing surface.

In the idealized situation where viscosity is neglected, the no-slip condition at the surface of the wing section does not apply.

Also in the absence of vorticity (inviscid flow) flow separation cannot occur.

Viscous flow around a stationary wing section:

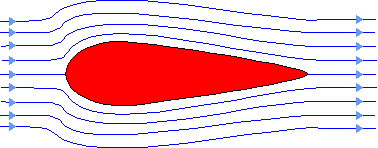One stagnation point is created in the wing section's leading edge.

Because of the viscosity a no-slip condition exists everywhere on the surface of the profile i.e. the velocity must vanish everywhere on the surface.

Consequently a boundary layer is created.

The inertia of the fluid as it rounds the top and bottom of the trailing surface causes the flow to separate at these locations.

This creates a disturbed wake that induces vortices downstream from the wing section.

Funded in part by

Updated: February 23, 1999